数学物理方程具有很强的物理背景,代表了前电脑时代人类的最高智慧水平,觉得难是很正常的。代写之家数学代考、 物理代考、exam代考、 线上代考、online test、take homework 服务。基础物理声光电磁学,理论物理量子力学,经典力学,电动力学,统计力学等,数学离散数学、数理统计、抽象代数、微分方程、测度论、实变函数、数学物理方程等代考代写
在很多大学,数学物理方程代写这门课程是数学专业和物理专业学生混合在一起上课的。
数学物理方程了,方程自然是从物理里面来的,背景也都是些物理背景(当然也有不是源于物理的方程,同样的方程也有很多解释的方式)。这门课的主要目的是教你解方程,因此大多数老师只会简略地分析一下方程的物理背景,或者干脆留给学生自己看,而数学出身的作者编的教材关于物理背景的部分基本上也都是写得能看的样子就算了。你要是数学系的学生觉得这些背景费解,一开始直接跳过都没啥太大的问题,后面再慢慢想。你要是强迫症,非得搞清楚一点,先学学物理或许好一点。各种微分方程千千万,其中最具有代表性的是三个:波动方程,热传导方程,拉普拉斯/泊松方程(也叫场方程)。
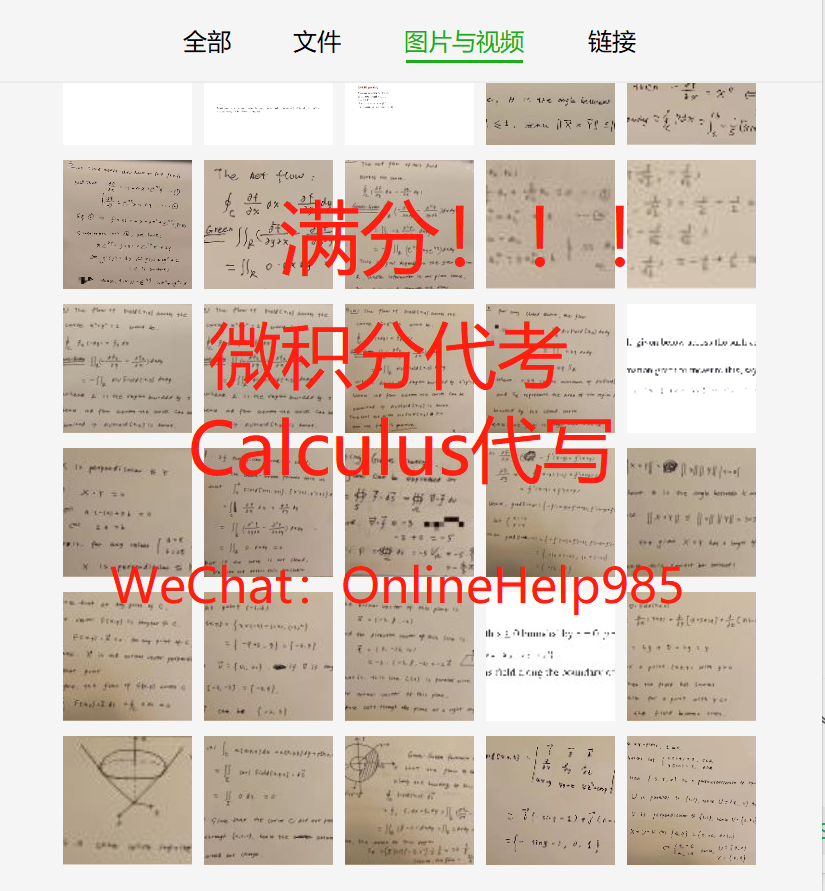
下面是一则数学物理方程作业的实战
Question 1)
(a) Show that, by choosing suitable values for P and Q, Green’s Theorem in the
plane leads to the formula for the area enclosed by a loop i.e.,
Area =
1
2 ˛loop
xdy − ydx
[2 marks]
(b) Use this to find the area of the closed curve defined by
x = cos �
y = 3 sin �
where 0 ≤ � ≤ 2�. [4 marks]
Question 2)
Consider the vector field, F = x2yz i+xy2z j+xyz2 k. Use the divergence theorem
to evaluate
” F · ds
over the surface of the unit cube defined by the ranges x = [0, 1]; y = [0, 1]; z = [0, 1].
[4 marks]
Question 3)
Consider the vector field F = (2x+yz) i+(2y+xz) j+xyk. Using Stokes’ theorem,
show that ¸ F · dr = 0 around any closed curve. [2 marks]
Question 4)
Consider the vector field, F = y i+x k. Use Stokes’ theorem to find ¸ F·dr around
the circular loop in the xy-plane defined by x2 + y2 = a2. [4 marks]
Question 5)
For gravity, g, we can define the divergence as, ∇ · g = −4�G�, where G is the
gravitational constant and � is the mass density. The divergence theorem states that,
“S
g · ds = °V
∇ · g dV.
By applying the divergence theorem to a point within the Earth’s radius, a distance
r from the centre:
(a) Draw a sketch showing a suitable choice of surface, S. [2 marks]
(b) Show that the magnitude of g is given by:
g(r) = −
4�
3
G�r
(Assume that the Earth has a constant density.) [2 marks]
Question 6)
The square OABC lies in the xy-plane and is defined by the points:
O = (0, 0, 0);A = (1, 0, 0);B = (1, 1, 0);C = (0, 1, 0). The vector field, b, is given by,
b = 2yz i + (x2 − y2) j + (y + x2 − z2) k.
(a) Evaluate the line integral, I1 = ¸ b · dr, following the path O-A-B-C-O.
[4 marks]
(b) Determine ∇ × b. [2 marks]
(c) Evaluate, I2 = ˜OABC(∇ × b) · ds, over the square OABC. [4 marks]
代写之家为海外留学生提供全方面的线上辅导,包括但不限于:exam代考、留学生考试、代考, 作业代写,留学生作业代写, 网课代上、网课代考,致力于提升Exam/Quiz分数并向更高GPA冲刺。同时提供考试辅助等在线解题服务。